SELINA Solutions for Class 9 Maths Chapter 28 - Distance Formula

 

Chapter 28 - Distance Formula Exercise Ex. 28

Question 1

Find the distance between the following pairs of points:

(i) (-3, 6) and (2, -6)

(ii) (-a, -b) and (a, b)

(iii)  and 

(iv)  and 

Solution 1

(i) (-3, 6) and (2, -6)

Distance between the given points

 

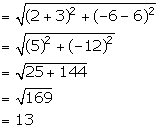
(ii) (-a, -b) and (a, b)

Distance between the given points

 

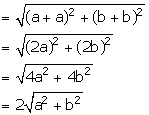
(iii)  and 

Distance between the given points

 

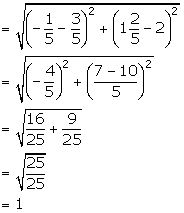
(iv)  and 

Distance between the given points

Question 2

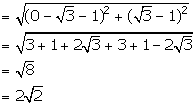
Find the distance between the origin and the point:

(i) (-8, 6) (ii) (-5, -12) (iii) (8, -15)

Solution 2

Coordinates of origin are O (0, 0).

(i) A (-8, 6)

AO = 

 

(ii) B (-5, -12)

BO = 

 

(iii) C (8, -15)

CO = 

Question 3

The distance between the points (3, 1) and (0, x) is 5. Find x.

Solution 3

It is given that the distance between the points A (3, 1) and B (0, x) is 5.

Question 4

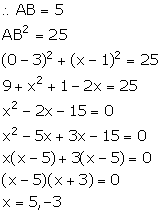
Find the co-ordinates of points on the x-axis which are at a distance of 17 units from the point (11, -8).

Solution 4

Let the coordinates of the point on x-axis be (x, 0).

From the given information, we have:

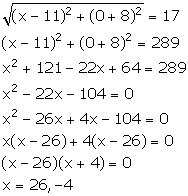
Thus, the required co-ordinates of the points on x-axis are (26, 0) and (-4, 0).

Question 5

Find the co-ordinates of the points on the y-axis, which are at a distance of 10 units from the point (-8, 4).

Solution 5

Let the coordinates of the point on y-axis be (0, y).

From the given information, we have:

Thus, the required co-ordinates of the points on y-axis are (0, 10) and (0, -2).

Question 6

A point A is at a distance of  unit from the point (4, 3). Find the co-ordinates of point A, if its ordinate is twice its abscissa.

Solution 6

It is given that the co-ordinates of point A are such that its ordinate is twice its abscissa.

So, let the co-ordinates of point A be (x, 2x).

We have:

Thus, the co-ordinates of the point A are (1, 2) and (3, 6).

Question 7

A point P (2, -1) is equidistant from the points (a, 7) and (-3, a). Find a.

Solution 7

Given that the point P (2, -1) is equidistant from the points A (a, 7) and B (-3, a).

Question 8

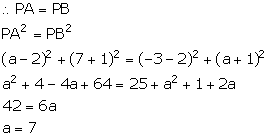
What point on the x-axis is equidistant from the points (7, 6) and (-3, 4)?

Solution 8

Let the co-ordinates of the required point on x-axis be P (x, 0).

The given points are A (7, 6) and B (-3, 4).

Given, PA = PB

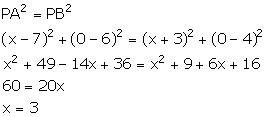
Thus, the required point is (3, 0).

Question 9

Find a point on the y-axis which is equidistant from the points (5, 2) and (-4, 3).

Solution 9

Let the co-ordinates of the required point on y-axis be P (0, y).

The given points are A (5, 2) and B (-4, 3).

Given, PA = PB

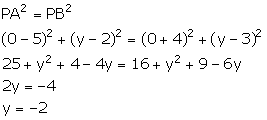
Thus, the required point is (0, -2).

Question 10

A point P lies on the x-axis and another point Q lies on the y-axis.

(i) Write the ordinate of point P.

(ii) Write the abscissa of point Q.

(iii) If the abscissa of point P is -12 and the ordinate of point Q is -16; calculate the length of line segment PQ.

Solution 10

(i) Since, the point P lies on the x-axis, its ordinate is 0.

(ii) Since, the point Q lies on the y-axis, its abscissa is 0.

(iii) The co-ordinates of P and Q are (-12, 0) and (0, -16) respectively.

 

PQ = 

Question 11

Show that the points P (0, 5), Q (5, 10) and R (6, 3) are the vertices of an isosceles triangle.

Solution 11

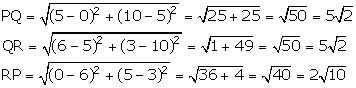
Since, PQ = QR, PQR is an isosceles triangle.

Question 12

Prove that the points P(0, -4), Q(6, 2), R(3, 5) and S(-3, -1) are the vertices of a rectangle PQRS.

Solution 12

Question 13

Prove that the points A (1, -3), B (-3, 0) and C (4, 1) are the vertices of an isosceles right-angled triangle. Find the area of the triangle.

Solution 13

Question 14

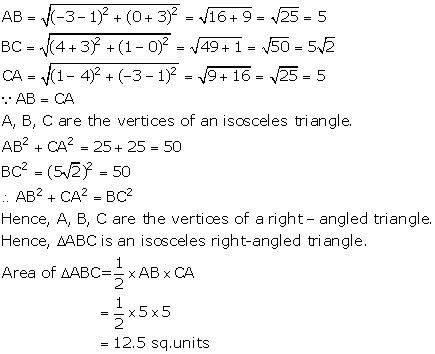
Show that the points A (5, 6), B (1, 5), C (2, 1) and D (6, 2) are the vertices of a square ABCD.

Solution 14

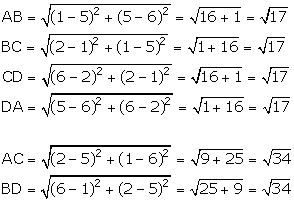
Since, AB = BC = CD = DA and AC = BD,

A, B, C and D are the vertices of a square.

Question 15

Show that (-3, 2), (-5, -5), (2, -3) and (4, 4) are the vertices of a rhombus.

Solution 15

Let the given points be A (-3, 2), B (-5, -5), C (2, -3) and D (4, 4).

 

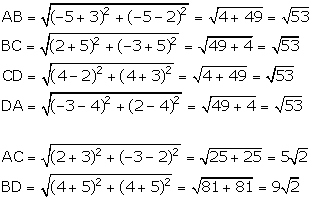
Since, AB = BC = CD = DA and AC  BD

The given vertices are the vertices of a rhombus.

Question 16

Points A (-3, -2), B (-6, a), C (-3, -4) and D (0, -1) are the vertices of quadrilateral ABCD; find a if 'a' is negative and AB = CD.

Solution 16

AB = CD

AB² = CD²

(-6 + 3)² + (a + 2)² = (0 + 3)² + (-1 + 4)²

9 + a² + 4 + 4a = 9 + 9

a² + 4a - 5 = 0

a² - a + 5a - 5 = 0

a(a - 1) + 5 (a - 1) = 0

(a - 1) (a + 5) = 0

a = 1 or -5

 

It is given that a is negative, thus the value of a is -5.

Question 17

The vertices of a triangle are (5, 1), (11, 1) and (11, 9). Find the co-ordinates of the circumcentre of the triangle.

Solution 17

Let the circumcentre be P (x, y).

Then, PA = PB

PA² = PB²

(x - 5)² + (y - 1)² = (x - 11)² + (y - 1)²

x² + 25 - 10x = x² + 121 - 22x

12x = 96

x = 8

 

Also, PA = PC

PA² = PC²

(x - 5)² + (y - 1)² = (x - 11)² + (y - 9)²

x² + 25 - 10x + y² + 1 - 2y = x² + 121 - 22x + y² + 81 - 18y

12x + 16y = 176

3x + 4y = 44

24 + 4y = 44

4y = 20

y = 5

 

Thus, the co-ordinates of the circumcentre of the triangle are (8, 5).

Question 18

Given A = (3, 1) and B = (0, y - 1). Find y if AB = 5.

Solution 18

AB = 5

AB² = 25

(0 - 3)² + (y - 1 - 1)² = 25

9 + y² + 4 - 4y = 25

y² - 4y - 12 = 0

 

y² - 6y + 2y - 12 = 0

y(y - 6) + 2(y - 6) = 0

(y - 6) (y + 2) = 0

y = 6, -2

Question 19

Given A = (x + 2, -2) and B (11, 6). Find x if AB = 17.

Solution 19

AB = 17

AB² = 289

(11 - x - 2)² + (6 + 2)² = 289

x² + 81 - 18x + 64 = 289

x² - 18x - 144 = 0

x² - 24x + 6x - 144 = 0

x(x - 24) + 6(x - 24) = 0

(x - 24) (x + 6) = 0

x = 24, -6

Question 20

The centre of a circle is (2x - 1, 3x + 1). Find x if the circle passes through (-3, -1) and the length of its diameter is 20 unit.

Solution 20

Distance between the points A (2x - 1, 3x + 1) and B (-3, -1) = Radius of circle

AB = 10 (Since, diameter = 20 units, given)

AB² = 100

(-3 - 2x + 1)² + (-1 - 3x - 1)² = 100

(-2 - 2x)² + (-2 - 3x)² = 100

4 + 4x² + 8x + 4 + 9x² + 12x = 100

13x² + 20x - 92 = 0

Question 21

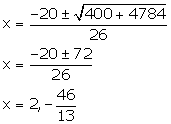
The length of line PQ is 10 units and the co-ordinates of P are (2, -3); calculate the co-ordinates of point Q, if its abscissa is 10.

Solution 21

Let the co-ordinates of point Q be (10, y).

PQ = 10

PQ² = 100

(10 - 2)² + (y + 3)² = 100

64 + y² + 9 + 6y = 100

y² + 6y - 27 = 0

y² + 9y - 3y - 27 = 0

y(y + 9) - 3(y + 9) = 0

(y + 9) (y - 3) = 0

y = -9, 3

 

Thus, the required co-ordinates of point Q are (10, -9) and (10, 3).

Question 22

Point P (2, -7) is the centre of a circle with radius 13 unit, PT is perpendicular to chord AB and T = (-2, -4); calculate the length of:

(i) AT (ii) AB.

 

Solution 22

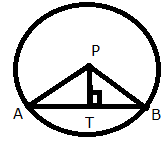
(i) Given, radius = 13 units

PA = PB = 13 units

 

Using distance formula,

 

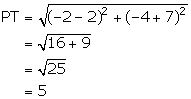
Using Pythagoras theorem in PAT,

AT² = PA² - PT² = 169 - 25 = 144

AT = 12 units

 

(ii) We know that the perpendicular from the centre of a circle to a chord bisects the chord.

AB = 2AT = 2  12 units = 24 units

Question 23

Calculate the distance between the points P (2, 2) and Q (5, 4) correct to three significant figures.

Solution 23

Question 24

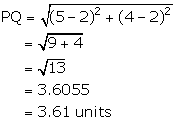
Calculate the distance between A (7, 3) and B on the x-axis whose abscissa is 11.

Solution 24

We know that any point on x-axis has coordinates of the form (x, 0).

Abscissa of point B = 11

Since, B lies of x-axis, so its co-ordinates are (11, 0).

Question 25

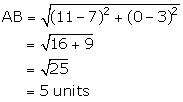
Calculate the distance between A (5, -3) and B on the y-axis whose ordinate is 9.

Solution 25

We know that any point on y-axis has coordinates of the form (0, y).

Ordinate of point B = 9

Since, B lies of y-axis, so its co-ordinates are (0, 9).

Question 26

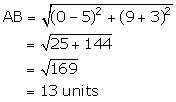
Find the point on y-axis whose distances from the points A (6, 7) and B (4, -3) are in the ratio 1: 2.

Solution 26

Let the required point on y-axis be P (0, y).

 

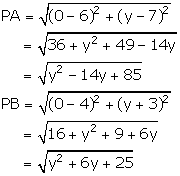
From the given information, we have:

 

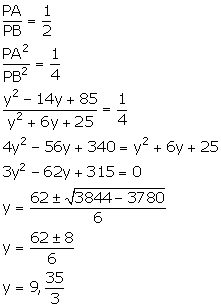
Thus, the required points on y-axis are (0, 9) and.

Question 27

The distances of point P (x, y) from the points A (1, -3) and B (-2, 2) are in the ratio 2: 3. Show that: 5x² + 5y² - 34x + 70y + 58 = 0.

Solution 27

It is given that PA: PB = 2: 3

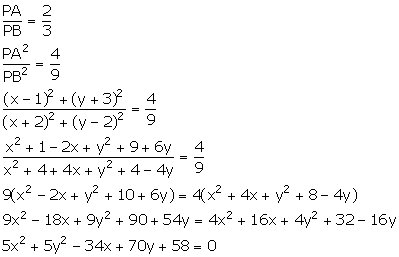
Hence, proved.

Question 28

The points A (3, 0), B (a, -2) and C (4, -1) are the vertices of triangle ABC right angled at vertex A. Find the value of a.

Solution 28

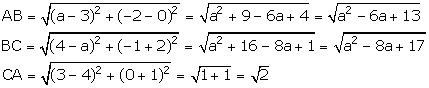
Since, triangle ABC is a right-angled at A, we have:

AB² + AC² = BC²

 a² - 6a + 13 + 2 = a² - 8a + 17

 2a = 2

 a = 1